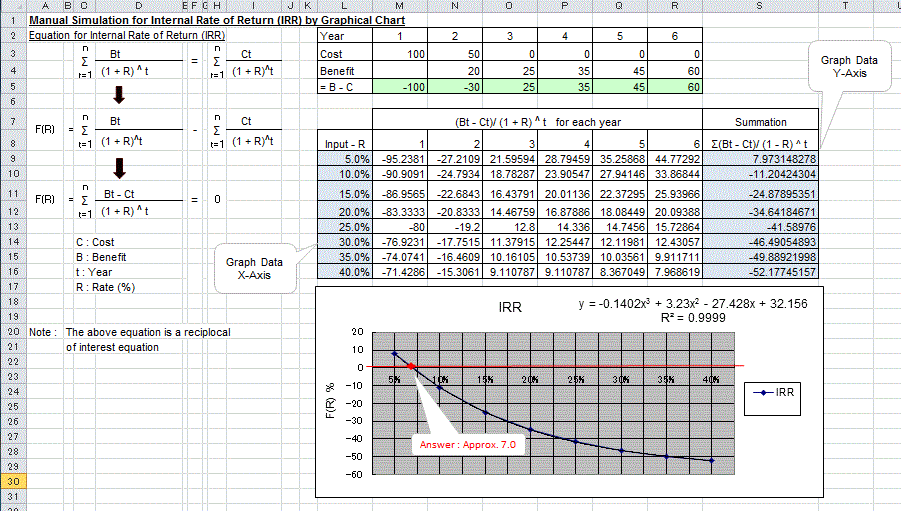
Equation of Internal Rate of Return (IRR) is referred to the left of Spreadsheet below.
The right table displays the graphical technique to make a curve of F(R) per IRR guess values.
F(R) is the net present value as a function of IRR.
The root of function is IRR at F(R)=0, then IRR is estimated approximately at 7 in the graphic chart.
Cell (M9) contains formula of "=M$5/(1+$L9)^M$8"
Columns "J"-"K": Newton Equation for IRR
Columns "N"-"X": Iterated Calculation by Formuls
Then, IRR= 0.0688 means 6.88% at Deviation=0.0%
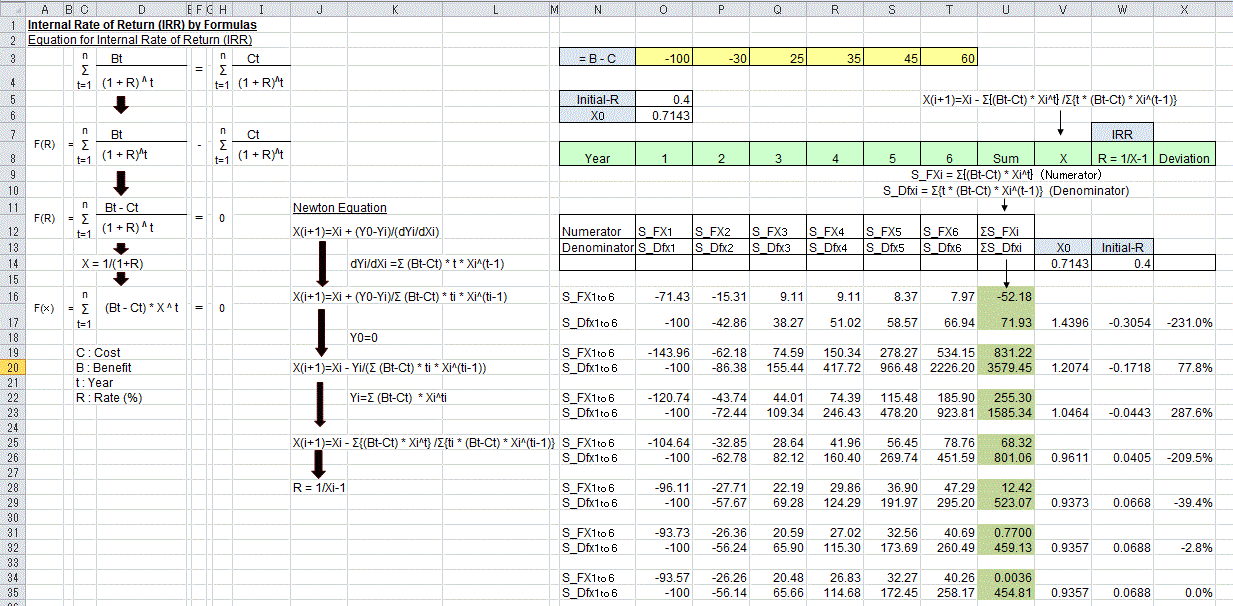
The sample IRR calculation is based on the Newton–Raphson method and
the sample file has been revised with English help menu in the user defined function (UDF) and
additional Macro UDF with a difference method without preparation of differential equation
if it is impossible, difficult or tiresome to prepare the differential equation.
Download Sample File
This tequnique contains followins.
- Auto-numbering (1, 2, 3, ......)
- Auto-numbering in filtrated items
- Separate auto-numbering in each group
- Auto-group numbering (example, A class : 1, B class : 2 ....)
- Auto-numbering of Item per Shipment (Added)
- etc.
Download : Numbering Technique-r1